What is 2/12 + 7/11?
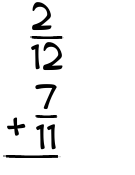
|
Here's how we add
|
||||||||||||||||||
Step 1We can't add two fractions with different denominators (the bottom number). So you need to get a common denominator - both bottom numbers need to match. To do this, you'll multiply the denominators times each other... but the numerators have to change, too. They get multiplied by the other term's denominator. So we multiply 2 by 11, and get 22, then we multiply 12 by 11 and get 132. Do the same for the second term. We multiply 7 by 12, and get 84, then multiply 12 by 11 and get 132. So now our fractions look like this:
|
||||||||||||||||||
Step 2Since our denominators match, we can add the numerators. 22 + 84 = 106 So the answer is:
|
||||||||||||||||||
Step 3Last of all, we need to simplify the fraction, if possible. Can it be reduced to a simpler fraction? To find out, we try dividing it by 2... Are both the numerator and the denominator evenly divisible by 2? Yes! So we reduce it:
So far so good... let's try to divide by that number again. No good. So next you try the next prime number, which is 3... No good. So next you try the next prime number, which is 5... No good. So next you try the next prime number, which is 7... No good. So next you try the next prime number, which is 11... No good. So next you try the next prime number, which is 13... No good. So next you try the next prime number, which is 17... No good. So next you try the next prime number, which is 19... No good. So next you try the next prime number, which is 23... No good. So next you try the next prime number, which is 29... No good. So next you try the next prime number, which is 31... No good. So next you try the next prime number, which is 37... No good. So next you try the next prime number, which is 41... No good. So next you try the next prime number, which is 43... No good. So next you try the next prime number, which is 47... No good. So next you try the next prime number, which is 53... No good. So next you try the next prime number, which is 59... No good. 59 is larger than 53. So we're done reducing. And we're done! Here's the final answer to 2/12 + 7/11
|