What is 1/14 + 2/4?

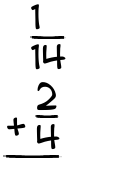
Here's how to add

Step 1

We still have different denominators (bottom numbers), though, so we need to get a common denominator. This will make the bottom numbers match. Multiply the denominators together first. Now, multiply each numerator by the other term's denominator.

Now we multiply 1 by 4, and get 4, then we multiply 14 by 4 and get 56.

Now for the second term. You multiply 2 by 14, and get 28, then multiply 14 by 4 and get 56.

This gives us a new problem that looks like so:

Step 2

Since our denominators match, we can add the numerators.

4 + 28 = 32

So what's the answer so far?

Step 3

Can this fraction be reduced?

First, we attempt to divide it by 2...

Are both the numerator and the denominator evenly divisible by 2? Yes! So we reduce it:

Let's try dividing by that again...

Are both the numerator and the denominator evenly divisible by 2? Yes! So we reduce it:

Let's try dividing by that again...

Are both the numerator and the denominator evenly divisible by 2? Yes! So we reduce it:

Let's try dividing by that again...

Nope! So now we try the next greatest prime number, 3...

Nope! So now we try the next greatest prime number, 5...

No good. 5 is larger than 4. So we're done reducing.

There you have it! Here's the final answer to 1/14 + 2/4